WOLFRAM NOTEBOOK

Kepler's Harmonices Mundi

​
Mercury
☿
0
octahedron
Venus
♀
0
icosahedron
Earth
0
dodecahedron
Mars
♂
0
tetrahedron
Jupiter
♃
0
cube
Saturn
♄
0
​
Harmony
of the World
In 1619 Johannes Kepler published Harmonices Mundi (The Harmony of the World). The book contains his definitive theory of the cosmos, blending a refined version of his original polyhedral theory with elliptic planetary orbits and the theory of musical harmony. Thanks to his harmonic law—nowadays the third law of planetary motion—he succeeded in fulfilling the ancient dream of proving that the heavens resound (silently) to the same chord and scale structures as Western music.
This Demonstration allows you to view and hear Kepler's universe. Tune the cosmos by placing the planets in the desired constellations by means of the sliders next to their names, or simply start from the great planetary alignment in Pisces, and click the "Harmony of the World" button to play and pause the music. By clicking the checkbox next to the planet names, you can include or exclude the tune of every single planet and display or hide the regular polyhedra and planetary spheres. The variation of a semitone in the tune of Mercury, a clink of the harpsichord, corresponds roughly to four terrestrial days.

Details

Kepler describes his cosmological theory "on the most perfect harmonies of the heavenly motions" in Book V of the Harmonices Mundi (1619)[1], after discussing the necessary geometric, harmonic, metaphysical and astrological prerequisites in Books I–IV. Book V opens with three chapters on his old polyhedral theory, developed 22 years earlier in the Mysterium Cosmographicum (1596). The remaining chapters combine this with his major astronomical achievements on elliptic planetary orbits, described in the Astronomia Nova (1609), and his musical theory, leading to his grand cosmic vision, the harmonic theory, also including his celebrated third law of planetary motion.
Kepler's Polyhedral Theory
Apparently, while lecturing on the periodic conjunction of Saturn and Jupiter, Kepler realized that if the radius of the insphere of an icosahedron is chosen equal to that of the circumsphere of an octahedron, then the radius of the insphere of a dodecahedron is chosen equal to that of the circumsphere of the icosahedron, and continuing with a tetrahedron and a cube, the six different radii of the inscribed and circumscribed spheres to the five regular polyhedra are in the same proportion as the radii of the orbits of the planets known at that time: Mercury, Venus, Earth, Mars, Jupiter and Saturn. Given that only five convex regular polyhedra exist,
Kepler interpreted this coincidence an a priori reason for the structure of the Copernican system. Later, the discrepancies between the prediction of this theory and those derived from the observation of the motions convinced Kepler that a refinement was needed. He introduced slightly different radii for the inspheres and circumspheres of the polyhedra to account for the elliptical orbits of the planets (see the diagram at[1, p. 405]).
Kepler's Harmonic Theory
The idea that the proportions in the motion of heavenly bodies are in agreement with those of musical harmony, known as harmony of the spheres or musica universalis (universal music), goes back as far as Pythagoras and remained a central theme of philosophical thinking for more than two millennia[2]. From Pythagoras (c. 570–495 BCE) to Plato (c. 420–347 BCE), Philolaus (c. 470–385 BCE), Cicero (106–43 BCE), Pliny (23–79 CE), Ptolemy (c. 100–170 CE), Boethius (c. 480–524 CE), Al Kindi (c. 801–873 CE), and Robert Fludd (1547–1637 CE), just to name the most influential of them, each scholar used different metaphysical or aesthetic arguments to associate to each celestial body a pitch, according to the best musical theory of his time[3]. On the contrary, Kepler (1571–1630 CE), who also made constant use of metaphysical arguments, proceeded in this case on a firm logical and quantitative ground. His constant search for harmony led him in 1618 to the discovery of his third planetary law. This associates a frequency to each planet in nearly uniform circular motion around the Sun. In the modern formulation
ν=
1
2π
GM
3
r
,
with
ν
the frequency,
G
the gravitational constant,
M
the solar mass and
r
the radius of the orbit. For example, the frequencies of the Earth at the aphelion and perihelion are respectively of about
3.02×
−8
10
Hz and
3.18×
−8
10
Hz, definitely outside the audible range. However, what matters in harmony is not the absolute pitch, but the relationship between two separate pitches. Since
3.18×
−8
10
/3.02×
−8
10
≃1.053
, Kepler could conclude that the "sounds" of the Earth at the aphelion and at the perihelion are separated by a semitone, like E and F. More generally, by using modern astronomical data we obtain the following:
​
aphelion
perihelion
​
ν(Hz)
ν
scaled
(Hz)
♩
ν(Hz)
ν
scaled
(Hz)
♩
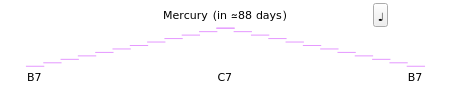
Mercury
9.71×
-8
10
2135
C7
1.82×
-7
10
3990
B7
Venus
4.98×
-8
10
1094
C6
5.12×
-8
10
1125
C6
Earth
3.02×
-8
10
664
F5
3.18×
-8
10
699
E5
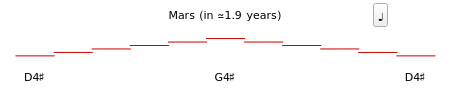
Mars
1.44×
-8
10
317
G4
1.89×
-8
10
418
D4
Jupiter
2.43×
-9
10
53
B1
2.81×
-9
10
62
A1
Saturn
9.71×
-10
10
21
G0
1.14×
-9
10
25
F0
where the pitches
ν
have been transposed to
ν
scaled
and associated to musical notes, so as to bring Saturn in the lowest audible range and the Earth at perihelion on E5. This allows Kepler to assert that "The Earth sings MI, FA, MI: you may infer even from the syllables that in this our home MIsery and FAmine hold sway." (marginalia on[1, p. 440]). Mi and Fa are the Latin names for E and F, respectively.
The planetary choir ranges over eight octaves, with the planets that "…advance from one extreme to the opposite one not by leaps and intervals, but with a continually changing note…"[1, p. 439]. However, Kepler "…could not express that in any other way but by a continuous series of intermediate notes"[1, p. 439]. Here we keep Kepler's discretization in semitones.
Mercury sings the "coloratura" as a treble and with an extension of an entire octave because of the great eccentricity of its orbit. Here it is rendered by a harpsichord (press ♩ to hear):
Venus and the Earth sing as alto. Due to the very small eccentricity of its orbit, Venus remains almost in unison, mumbling a single tune. The Earth sings instead misEry, Famine, misEry. Here they are rendered by two violins:
Mars, with an orbit of intermediate eccentricity, ranges over six semitones and sings the subject as a tenor. Here it is rendered by a viola:
Jupiter and Saturn, with eccentricities slightly bigger than Earth's, range over three semitones and sing as basso, modulating the "basso continuo". Here they are rendered by two cellos:
This unlikely baroque string quintet, accompanied by a harpsichord, continuously plays (silently) the Harmony of the World.

References

[1] J. Kepler, The Harmony of the World, (E. J. Aiton, A. M. Duncan and J. V. Field, trans.), Philadelphia: American Philosophical Society, 1997.
[2] B. Stephenson, The Music of the Heavens: Kepler's Harmonic Astronomy, Princeton, NJ: Princeton University Press, 1994.
[3] K. Ferguson, The Music of Pythagoras: How an Ancient Brotherhood Cracked the Code of the Universe and Lit the Path from Antiquity to Outer Space, New York: Walker & Co., 2008.

External Links

Permanent Citation

Wolfram Cloud

You are using a browser not supported by the Wolfram Cloud

Supported browsers include recent versions of Chrome, Edge, Firefox and Safari.


I understand and wish to continue anyway »

You are using a browser not supported by the Wolfram Cloud. Supported browsers include recent versions of Chrome, Edge, Firefox and Safari.