Lines
Euclid calls a line a “breadthless length”. In modern usage, a line is a primitive notion which become better defined by axioms. Euclidean, spherical, differential and projective geometries use different axiom sets. Euclid’s axiom that two points define a line via a straightedge construction classifies how lines operate in Euclidean geometry.
-
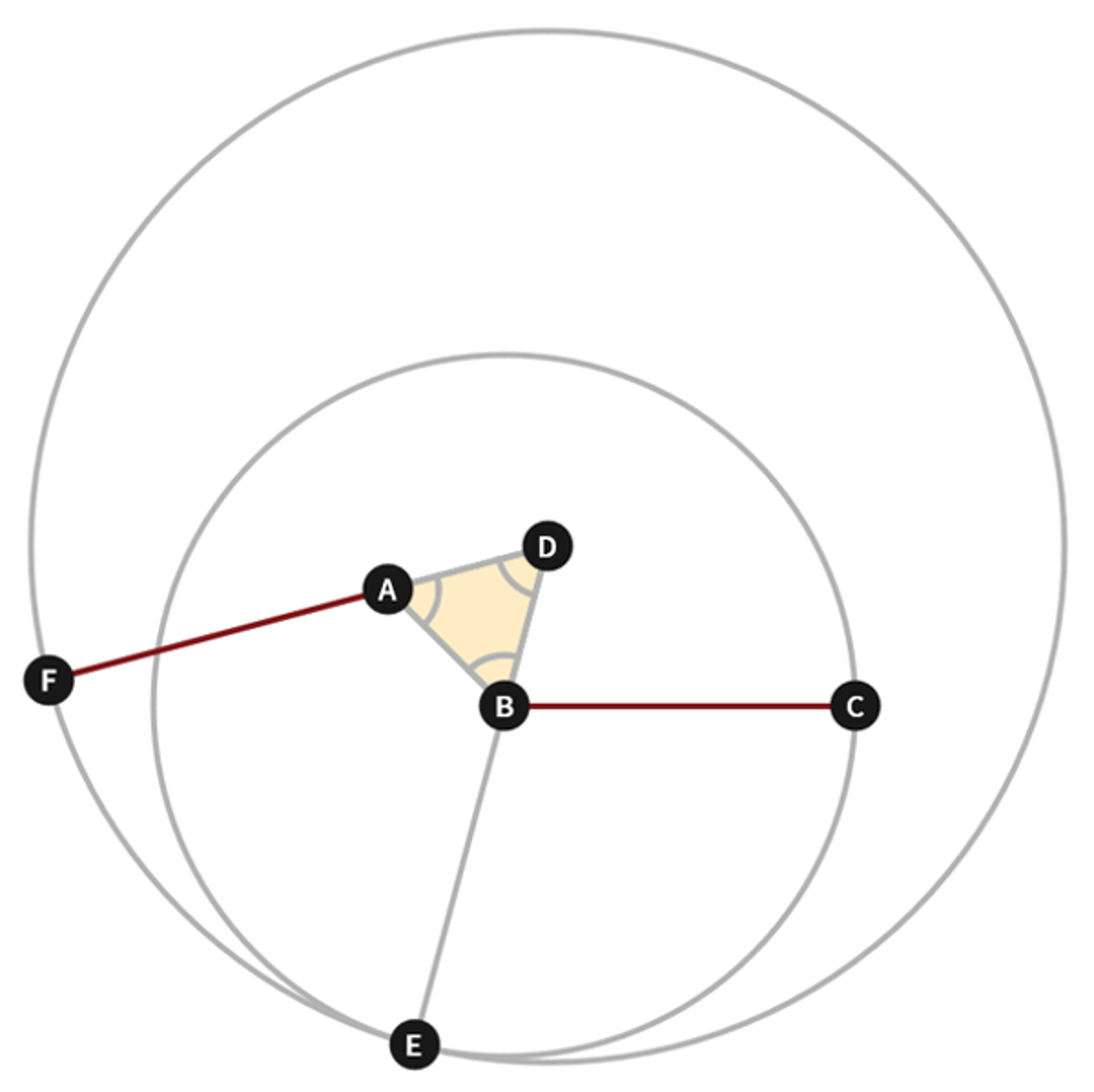
Euclid Book 1 Proposition 2
-
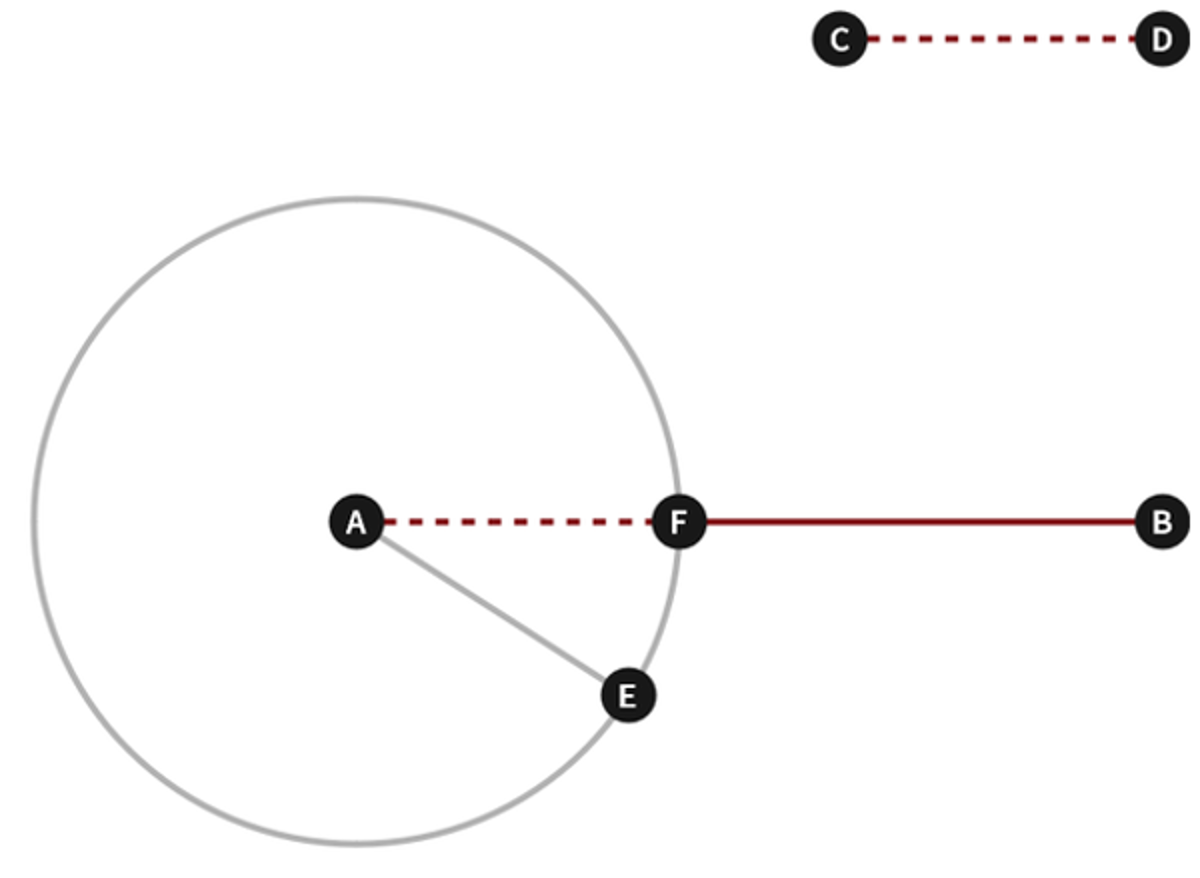
Euclid Book 1 Proposition 3
-
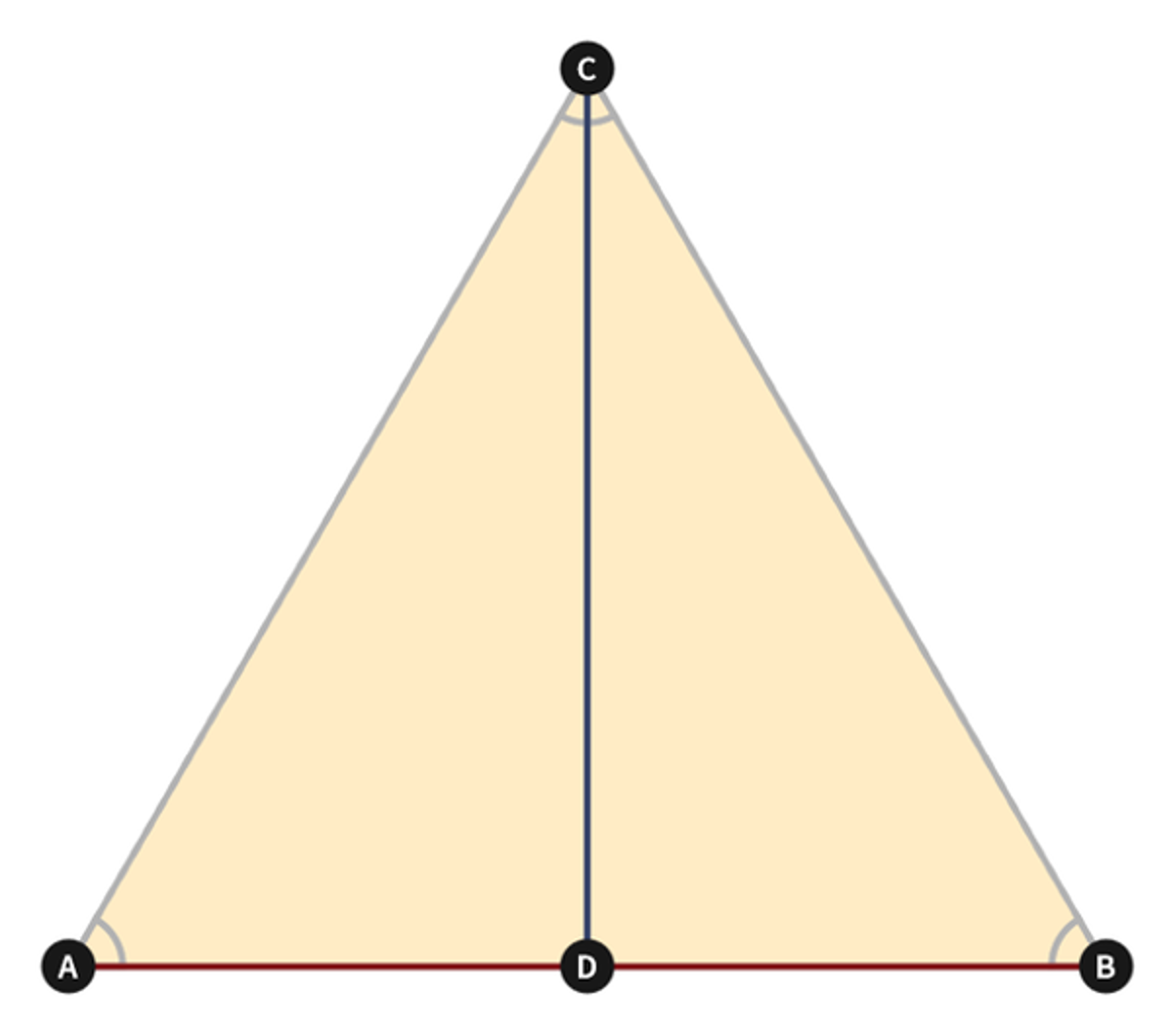
Euclid Book 1 Proposition 10
-
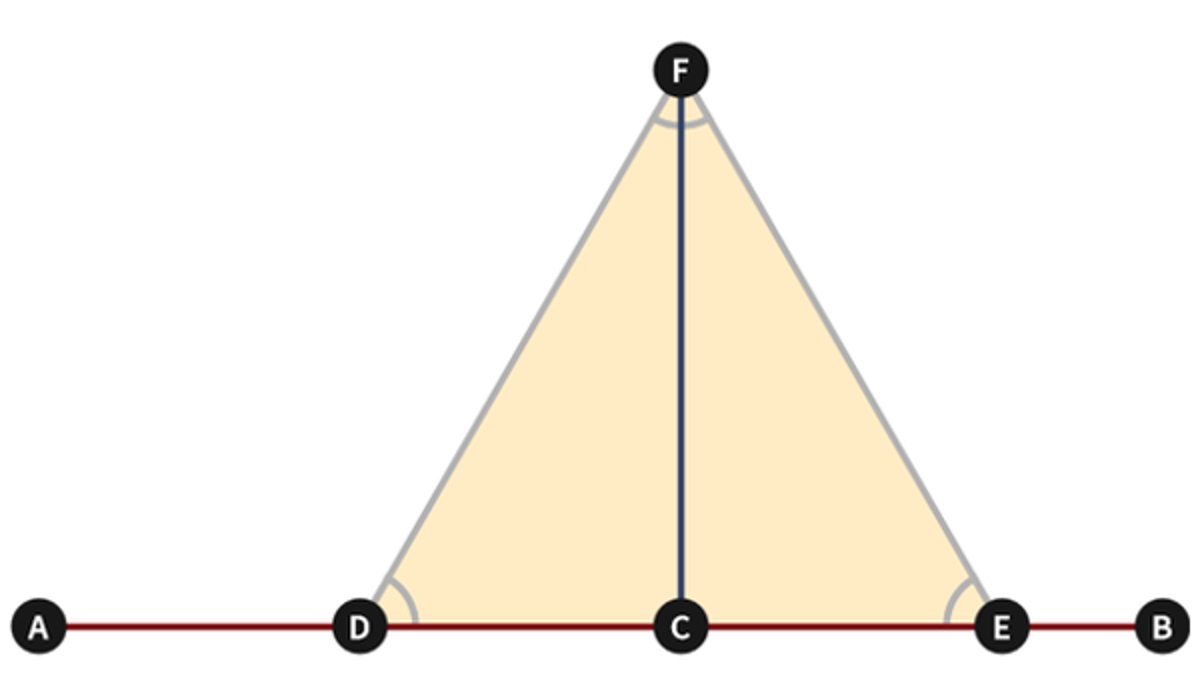
Euclid Book 1 Proposition 11
-
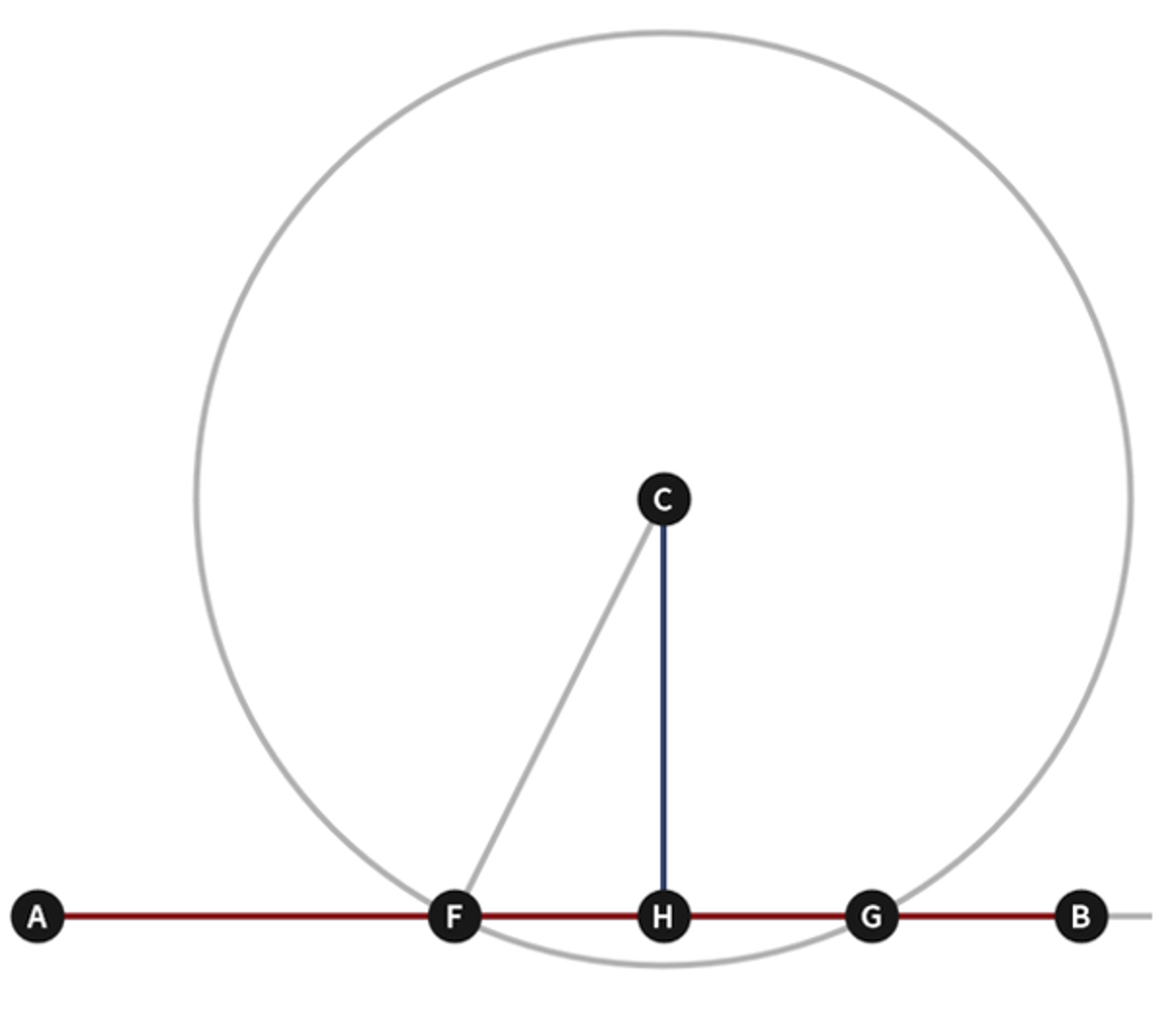
Euclid Book 1 Proposition 12
-
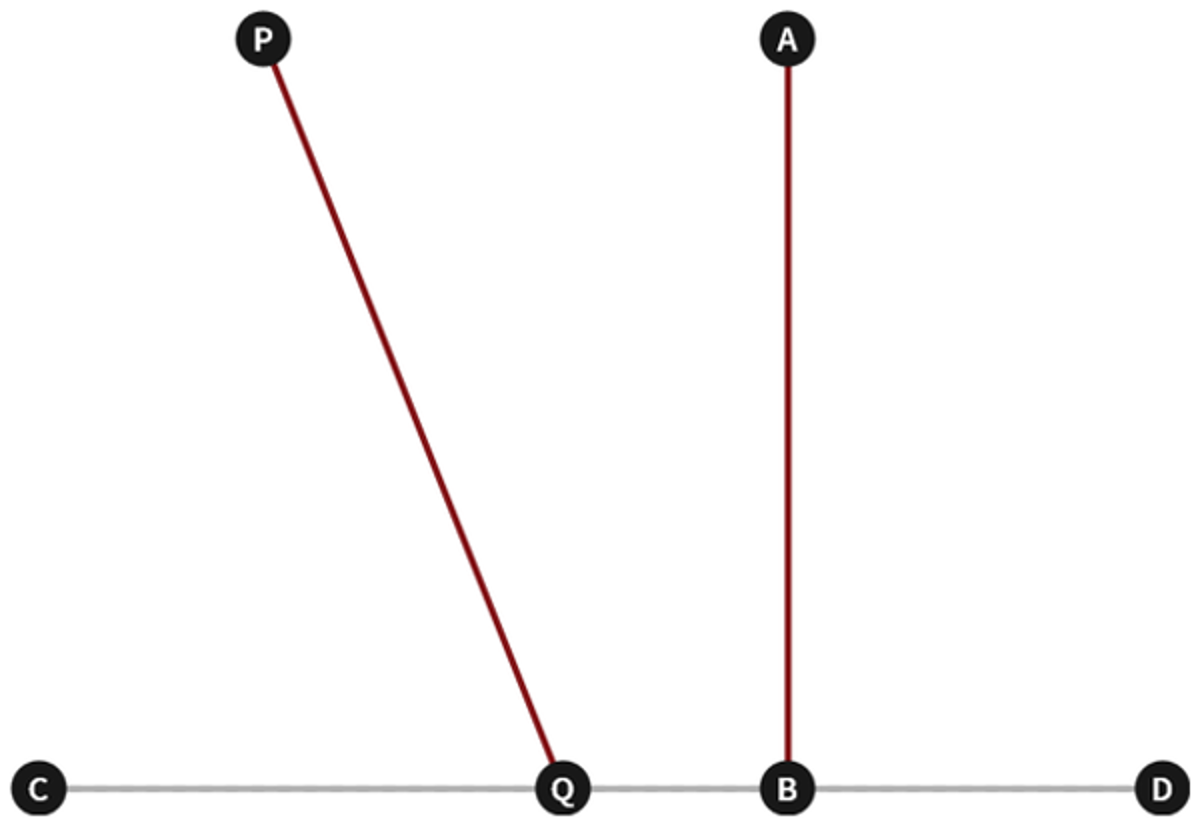
Euclid Book 1 Proposition 13
-
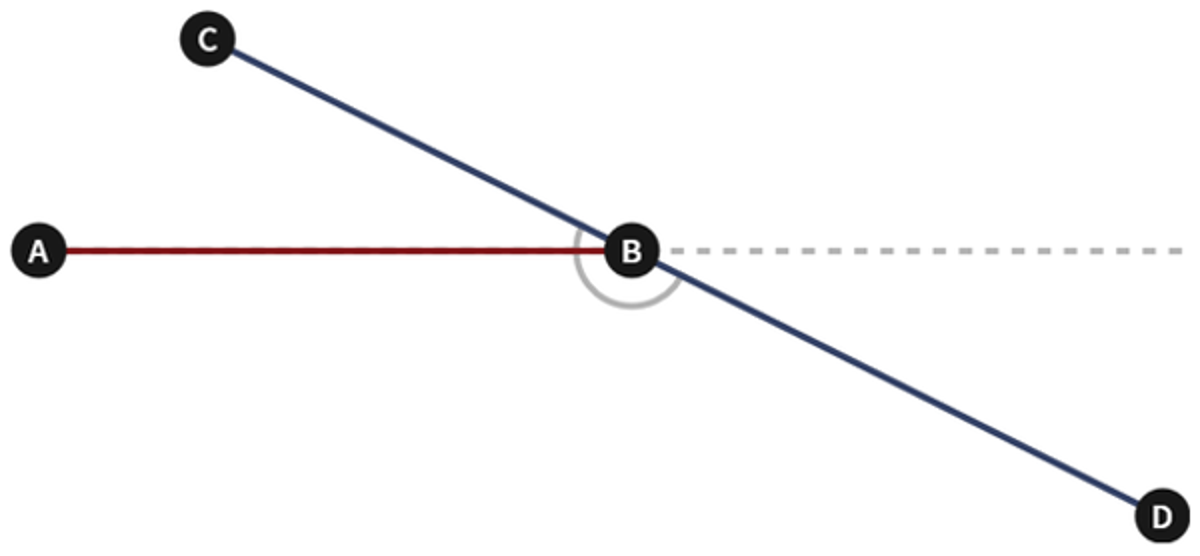
Euclid Book 1 Proposition 14
-

Euclid Book 1 Proposition 15
-
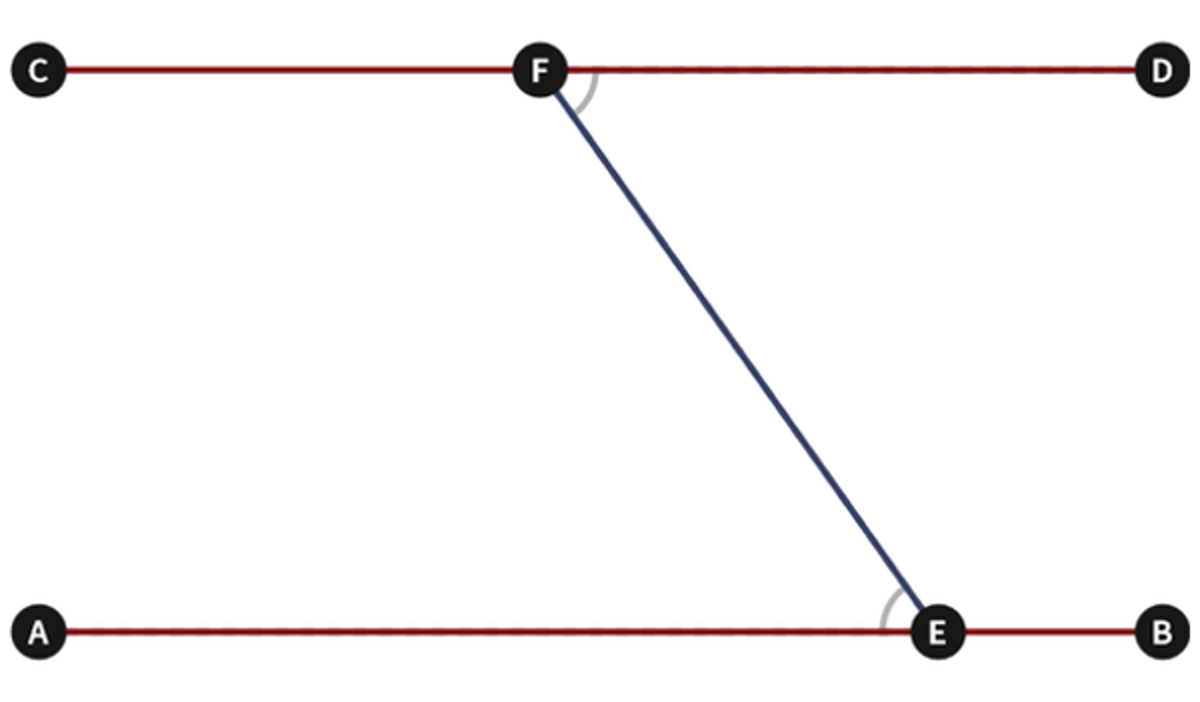
Euclid Book 1 Proposition 27
-
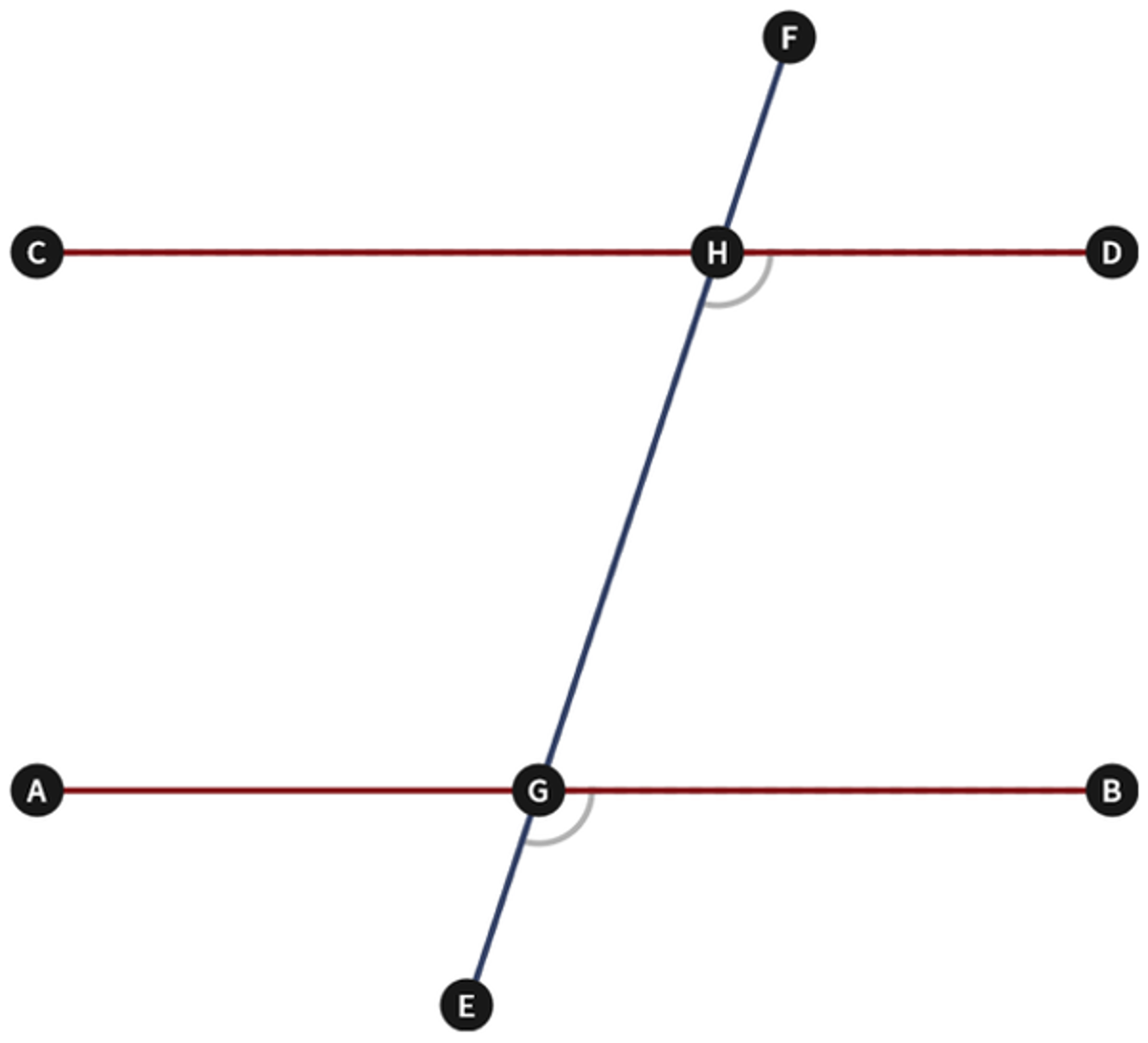
Euclid Book 1 Proposition 28a
-
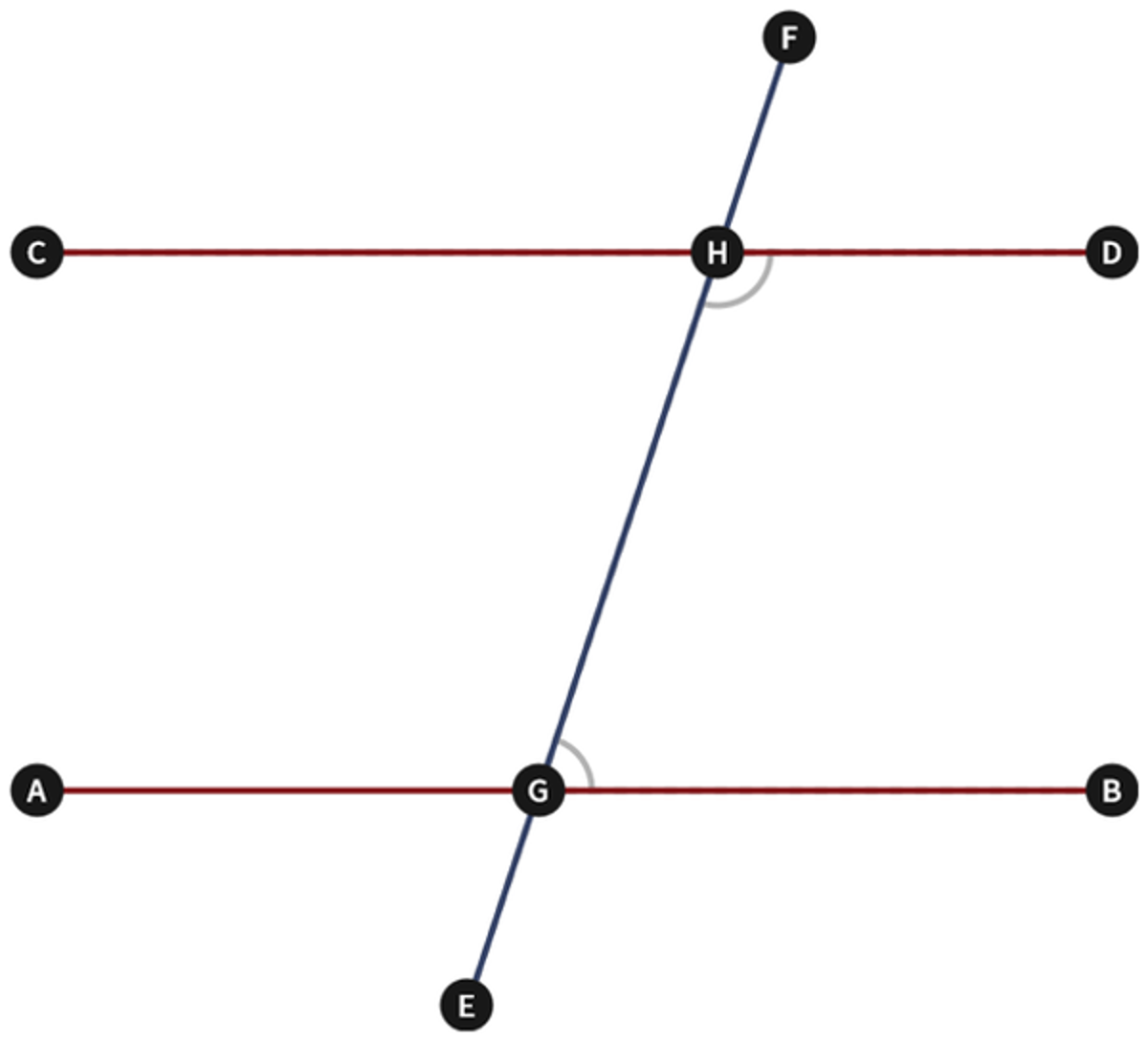
Euclid Book 1 Proposition 28b
-
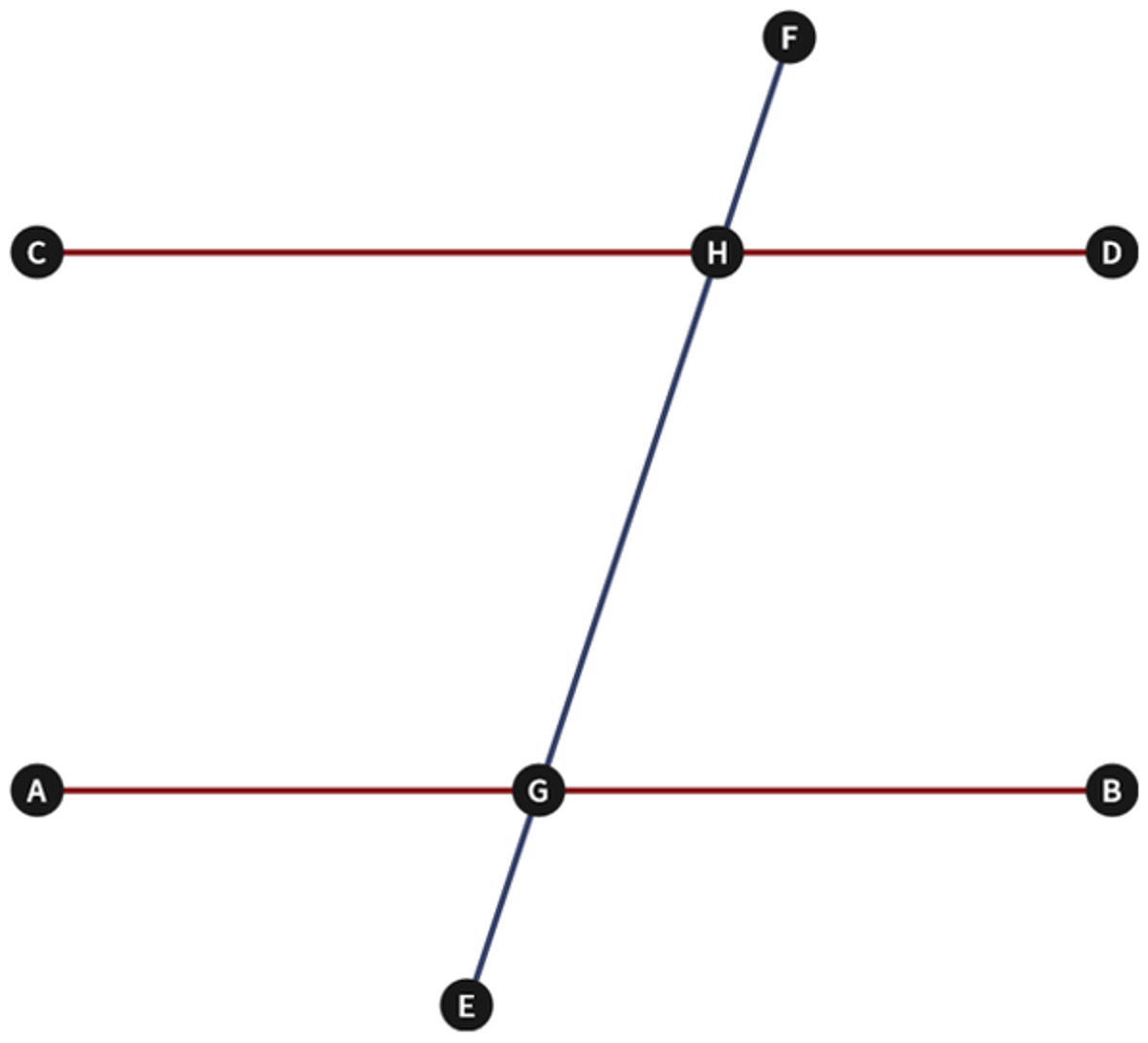
Euclid Book 1 Proposition 29
-
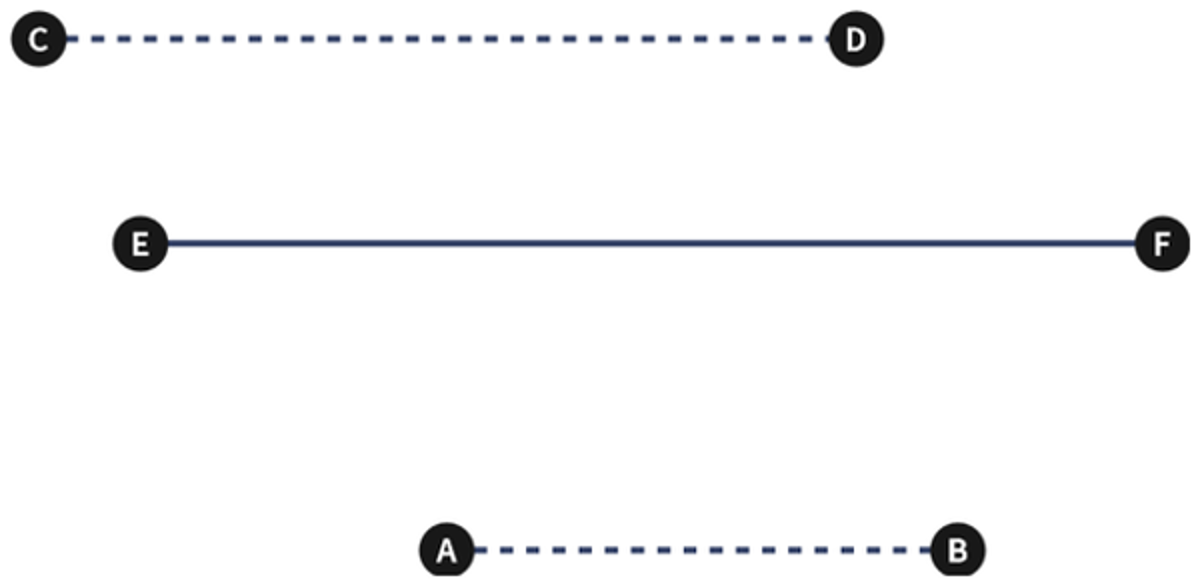
Euclid Book 1 Proposition 30
-
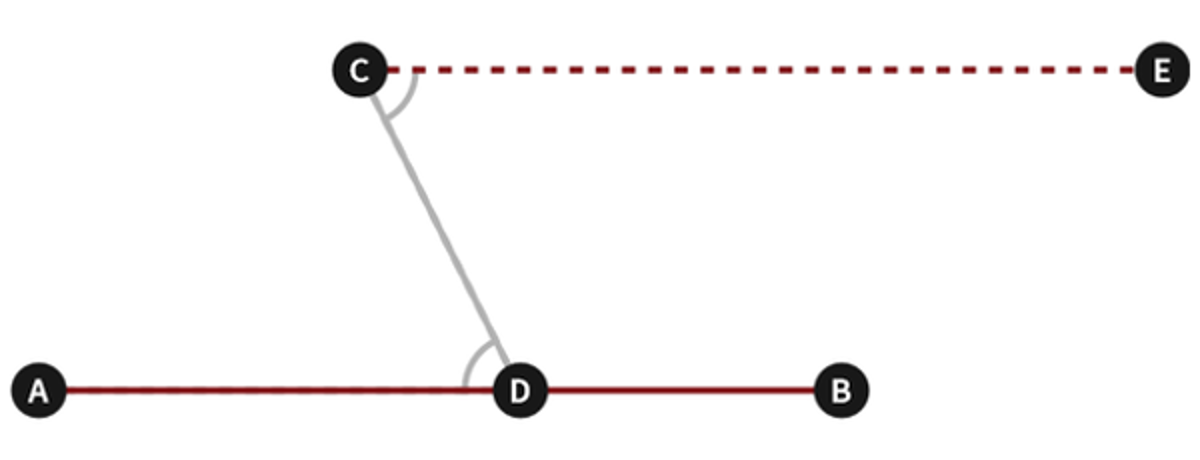
Euclid Book 1 Proposition 31
-
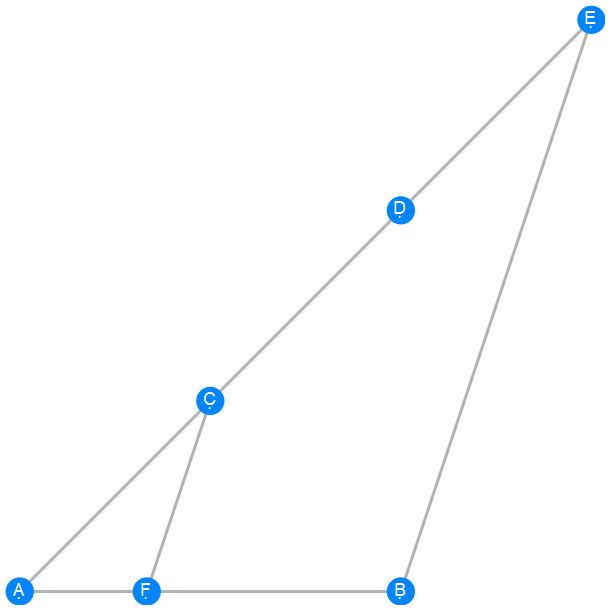
Euclid Book 6 Proposition 9
-
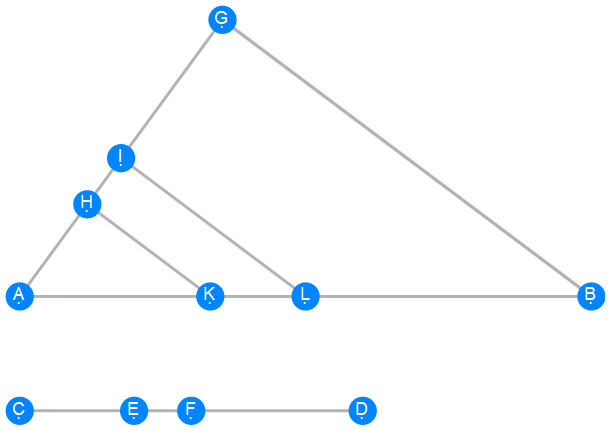
Euclid Book 6 Proposition 10
-
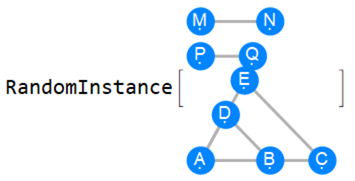
Euclid Book 6 Proposition 11
-
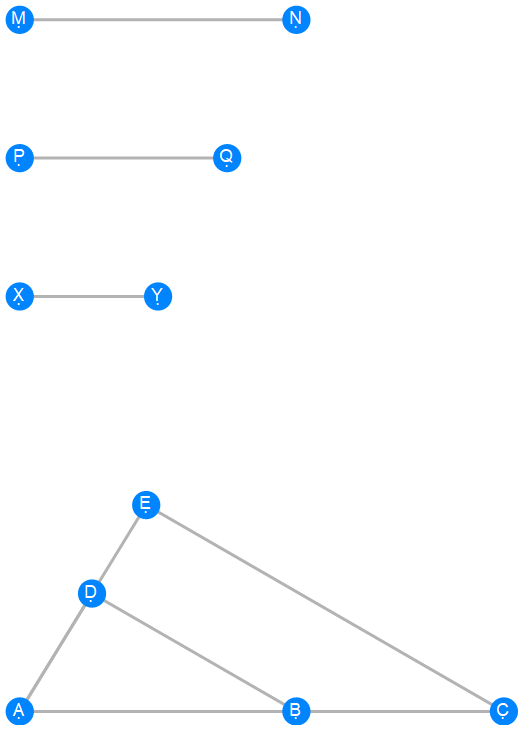
Euclid Book 6 Proposition 12
-
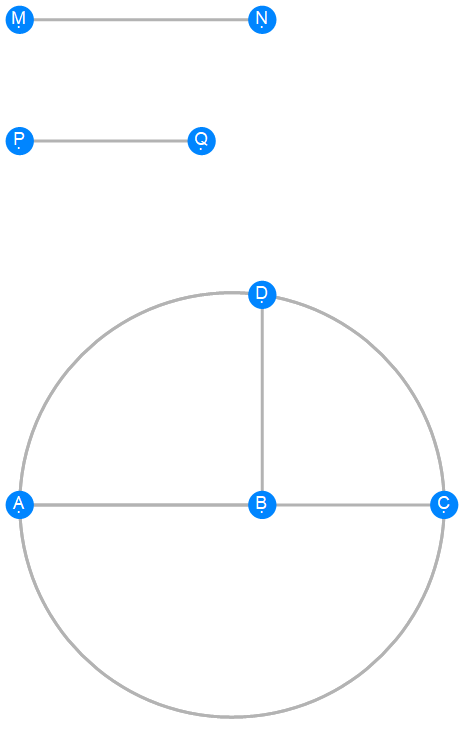
Euclid Book 6 Proposition 13
-
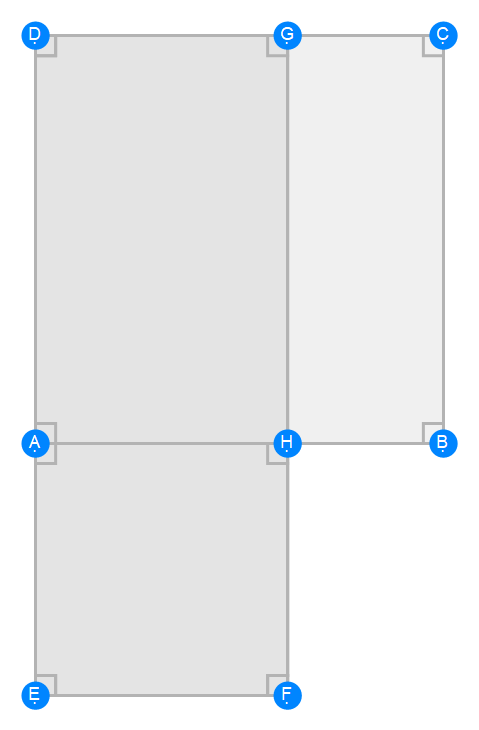
Euclid Book 6 Proposition 30