
You are using a browser not supported by the Wolfram Cloud
Supported browsers include recent versions of Chrome, Edge, Firefox and Safari.
I understand and wish to continue anyway »
Insert | Sample |


gtt[r,θ] | 0 | 0 | gtϕ[r,θ] |
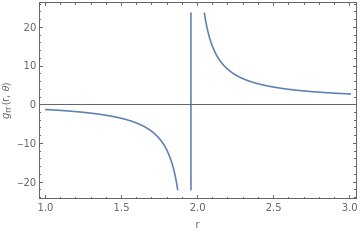
0 | grr[r,θ] | 0 | 0 |
0 | 0 | gθθ[r,θ] | 0 |
gtϕ[r,θ] | 0 | 0 | gϕϕ[r,θ] |

You are using a browser not supported by the Wolfram Cloud
Supported browsers include recent versions of Chrome, Edge, Firefox and Safari.
I understand and wish to continue anyway »